Take a look at the picture below:
 

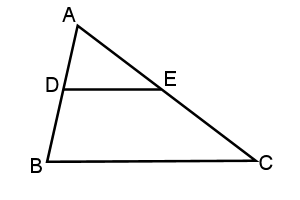
In this picture, DE is parallel to BC. <ADE and <ABC are corresponding angles, which means they are congruent, and similarly, <AED and <ACB are corresponding angles, so they also are congruent.

Since the two triangles (ADE and ABC) have two pairs of congruent angles, this means that they are similar. That makes sense, doesn't it? Just by looking at them you should be able to see that theylook similar, and we just proved that they are!

So let's see what we can do with this information...

Problem #1: If AD = 5, DB = 5, and DE = 6, what is the length of BC?

Solution: AD / AB = DE / BC. This gives us 5/10 = 6/BC, from which we conclude that BC = 12.

Problem #2: AB = 16, BC = 12, DE = 9. What is the length of DB?

Solution: AD / AB = DE / BC, or AD / 16 = 9 / 12, from which AD = 12. And since DB = AB - AD, DB = 16 - 12 = 4.

Problem #3: The perimeter of ABC is 40, and the perimeter of ADE is 10. If BC = 10, what is the length of DE?

Solution: The perimeters of the triangles are in the same ratio as the sides, so 10 / 40 = x / 10, and x = 2.5

For the sample problems, use the diagram to the left.

1.

AD is one third of DB, and BC is 27. What is the length of DE?

2.

If ABC is an equilateral triangle, and AD = 12, what is the length of DE?

3.

If <D is congruent to <E, DA = 10, and DB = 5 what is the length of AC?

4.

AD = 4 and AB = 10. If BC = 16, what is the length of DE?

5.

AD is two less than DB, BC is three more than DB, and DE is 3. What is the length of AD?

6.

DB is 4 more than AD, AE is twice AD, and EC = 14. What is the length of AB?

7.

If DB is one more than AD, AE is two more than AD, and EC is six more than AD, what is the length of AD?

8.

The lengths of the sides of triangle ABC are: AB= 10, BC = 12, and CA=16. If AD = 6, what are the lengths of DE and EA?

9.

If the perimeter of ABC is 24, and the perimeter of ADE is 16, and the length of AD is 3, what is the length of DB?

10.

AD = 12, DB = 8, and the perimeter of triangle ADE is 42, what is the perimeter of triangle ABC?