Introduction

Up to this point, we have considered motion of an object in either a straight line or a curved line as though the object had all its mass concentrated at one point. However, most objects in the real world have a physical size larger than zero, and in addition, in the motion of the object through space, we must consider the possibility that the object will rotate around some axis.

In studying rotation, we will proceed much as we did with linear motion. First we will learn how to describe rotation, a kind of rotational kinematics. We will then examine the causes of rotation, a kind of rotational dynamics. Finally we will apply our understanding of rotational motion to a special kind of motion called "Simple Harmonic Motion (SHM).

Angular Displacement

In the following discussion, refer to Figure 6.2.1 below. Consider an object which is free to rotate about some point, C, which we may call the axis of rotation. Let P be a second point located somewhere on the object other than the point C. The line drawn from C to P will be called the radius of the point P. Now draw a line, fixed in space, which we will refer to as the reference line. The angle between the reference line and the radius line will be labeled θ. This angle gives the angular position of the object with reference to the fixed line. It corresponds to the position of an object on the x axis, X, as discussed in the kinematics chapter.

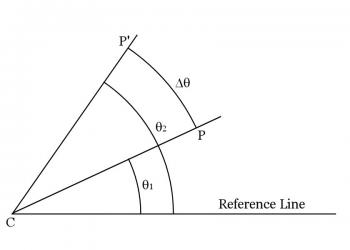
^{Figure 6.2.1}

Now let the object rotate around the point C. At one point in time the object might be located so its angular position is given by θ₁. At a later instant, it might be moved so its angular position is given by θ₂. The change in angular position is the difference between the two angles, labeled Δθ  (delta theta, or "change in theta") in the diagram.

Angular Velocity

We can now define an angular velocity much as we defined linear velocity. The symbol for angular velocity is usually a lower case omega: ω. Thus, we have:

The units of angular velocity are angle units divided by time units. You could use . However, it usually is simpler if you work in radian measurement and in this case angular velocity is measured in . Recall that in a complete circle there are 360º, or 2p radians.

Radian measure is preferred because of the ease with which you can go from linear measure to angular measure. Recall that for an arc of a circle, the arc length is equal to the radius of the circle multiplied by the number of radians in the angle which subtends the arc. Thus, the arc length for a complete circle (circumference) is the radius multiplied by the number of radians in a circle, 2p, or C = 2pr. Refer again to Figure 6.2.1. The distance, Δx, the point P has moved as the object rotated can be found by multiplying the angle through which the object has rotated (Δθ) by the radius.

If we divide both sides of Equation 2 by Δt, we obtain:

However, by definition, linear velocity =  and angular velocity = . Therefore, we find that:

In addition to the angular velocity, we often refer to the frequency of rotation. The frequency of rotation is the number of rotations an object completes in a particular unit of time, usually 1 second. It should be clear that since each complete circle contains 2p radians that the angular velocity and frequency are related as follows:

The period of rotation is defined as the time required to make one rotation. Suppose that an object rotates with a frequency of . It is apparent that it must take 0.1 sec to make one rotation. Thus, the relationship between frequency and period is

Angular Acceleration

If an object has its speed of rotation changing, it is possible to define an angular acceleration:

Using the same line of reasoning that we used in developing the relationship between angular and linear velocity we have:

Where a_t represents the tangential acceleration of the point P as the object rotates faster. Keep in mind that as with any object which is moving in a circular path, point P also has a central acceleration of .

Sample Problem

The flywheel of an engine has a diameter of 30cm and completes 3000 rotations each minute. Point P₁ is on the rim of the wheel and point P₂ is a point on the wheel which is 10cm from the center. Calculate:

1. the period of rotation
2. the angular velocity
3. the linear velocity of points P₁ and P₂
4. the central acceleration of the two points

Sample Solution

1.  Since the object rotates 3000 times each minute, it must rotate or 60 times per second. This is the frequency. The period is the reciprocal of the frequency.

2.  The angular velocity is the number of radians through which the object turns in one second. Since there are 2p radians in a circle, we have:

3.  There are two lines of reasoning to follow in determining the linear velocity. One is to say that the velocity is the distance traveled in one revolution divided by the time to complete one revolution. The distance traveled in one revolution is the circumference of the circle and the time for one revolution is the period. Thus we have:

4.  For central acceleration, use the velocities calculated in part 3

1.

The tip of a clock's hour hand travels as fast as the tip of the minute hand. If the minute hand is 15 cm long, how long is the hour hand?

2.

Express the angular speed of in revolutions per second.

3.

Express the angular speed of in revolutions per minute.

4.

Express the angular speed of in radians per second.

5.

Determine the angular speed of a car that travels around a curve of radius 25 ft at 30 mph (440 ).

6.

The circumferential speed of a 9 inch radius emery wheel is 1200 . Determine the angular speed of the wheel in revolutions per minute and in radians per second.