Forty-five and Thirty
Pro Problems > Math > Geometry > TrianglesForty-five and Thirty
Two of the angles of a triangle are 45 degrees and 30 degrees. The altitude from the third angle has length 15 units. What is the triangle's area?
Solution
In order to make it feasible for teachers to use these problems in their classwork, no solutions are publicly visible, so students cannot simply look up the answers. If you would like to view the solutions to these problems, you must have a Virtual Classroom subscription.Similar Problems
Angle Riddle
The angles of a triangle meet the following criteria:
- All the angle measures are prime numbers.
- All the angle measures are distinct.
- Exactly two of my angle measures are palindromic.
- The difference between my two largest angle measures is a two digit number x.
- One of the digits of x is twice the other digit.
Angles in a Triangle
In a triangle, the measure of the largest angle is 12 less than the sum of the measures of the other two angles. The largest angle is also 52 more than the twice the middle angle decreased by three times the smallest angle. What is the largest angle measure?
Triangle in a Square
If the perimeter of the triangle (in linear units) is equal to the area of the triangle (in square units), what is the length of the side of the square?
Split Triangle
Segment AD is drawn in triangle ABC, with D on side BC. Given the following information, determine the measure of angle ADB.
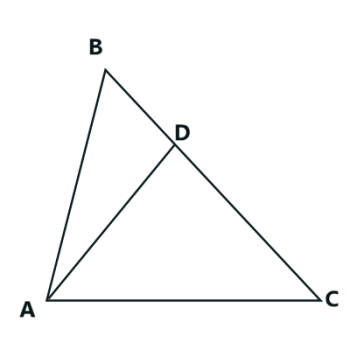
measure of angle BAD = x
measure of angle CAD = 2x
measure of angle ADC = 2y
measure of angle ABD = y
measure of angle ACD = x + y
Angle Measure
Line segment AB is intersected by segment DC, with D on AB, between A and B.
In triangle ADC, m∠ADC = 30º + x and m∠DAC = 50º
In triangle ABC, m∠ACB = 115º + x
In triangle CDB, m∠CDB = 140º + x
Find m∠CBD.
Quadratic Triangle
The angles in a triangle have measures x2 - 5, 2x + 18, and x + 37. What is the measure of the largest angle in the triangle?
Triangle in a Square
Triangle ABC is inside square ABDE, and C lies along side DE. If the area of the triangle is 24 square units, what is the length of AB?
Arithmetic Angles
The angles of a triangle are in arithmetic progression, and the difference between the largest and smallest angle is 42 degrees. What are the three angles?
Triangle and Rectangle
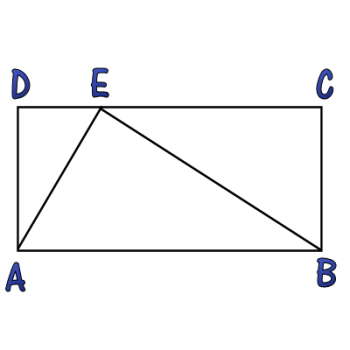
In the diagram displayed, the triangle splits the rectangle into three similar triangles. Find the value of AD2 divided by the product of DE and EC.
Angle Bisector
In the diagram shown,
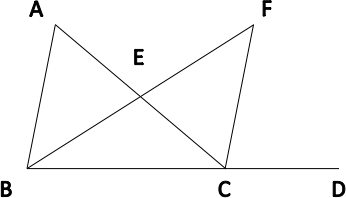
m ∠DCF = 80
m ∠ACF = 30
m ∠FBD = 50.
If segment BF bisects ∠ABD, find m ∠BAC.

